Quadratic Inequalities: A Step-by-Step Guide to Solving by Factorization

Calculator
Test Yourself

Quadratic Inequalities

  • Quadratic inequalities are mathematical statements that combine quadratic equations and an inequality symbol.

  • They are essential for students studying algebra, as they frequently appear in exams and real-life situations.

In this article, we will discuss: 

  1. What are Quadratic Inequalities?
  2. Quadratic Inequalities Solving by Factorization?

Here is one more link to practice a few extra questions: Maths Genie Quadratic Inequalities

What are Quadratic Inequalities?

  • A quadratic inequality can have one of three forms:

ax2 + bx + c < 0, ax2 + bx + c > 0

or

ax2 + bx + c ≤ 0, ax2 + bx + c ≥ 0

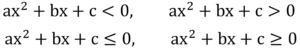
Quadratic Inequalities Formula

where a, b, and c are real numbers.

  • To solve quadratic inequalities by factorization, we need to follow these steps:
  1. Rewrite the inequality as an equation by replacing the inequality symbol with an equal sign.
  2. Factorize the quadratic equation.
  3. Determine the values of x that satisfy the equation.
  4. Use these values to solve the inequality.
Request for Demo Lesson

Quadratic Inequalities Solving by Factorization

  • Solving quadratic inequalities by factorisation is a common technique used to find the values of x that satisfy the inequality.
  • Here are the steps for solving a quadratic inequality using factorisation:

STEPS for Solving Quadratic Inequalities:

  • Step #1: Factorise the quadratic expression Start by factoring the quadratic expression on the left side of the inequality. For example, to solve the inequality

x2 – 4x + 3>0, we can factor it as (x – 1)(x – 3)>0

  • Step #2: Find the critical points The critical points are the values of x that make the left side of the inequality equal to zero. In this example, the critical points are

x = 1 and x = 3,

which are the roots of the factored quadratic expression.

  • Step #3: Plot the critical points on a number line Draw a number line and mark the critical points on it. In this example, we mark

x = 1 and x = 3

on the number line.

Quadratic Inequalities Number line

  • Step #4: To determine which intervals satisfy the inequality, you should test the intervals between the critical points.

    • In each interval, pick any value and substitute it into the original inequality.

    • If the inequality is true for that value, then the interval satisfies the inequality; otherwise, it does not.

    • For example, if you want to test the interval x < 1, you can pick a value like x = 0 and substitute it into the original inequality.

    • Calculate:

(0 – 1)(0 – 3), which results in (0 – 1)(0 – 3) > 0.

    • Since this inequality is true, it indicates that the interval x < 1 satisfies the inequality.

  • Step #5: Write the solution set Finally, we can write the solution set for the inequality.
    • In this example, the solution set is

x<1 or x>3.

certified Physics and Maths tutor

 

 

Solved Example: Quadratic Inequalities

Question 1:Solve the inequality 2x2 – 5x + 3 < 0 by factorisation.

Solution:

  • Step #1: Factorise the quadratic expression.

2x2 – 5x + 3 = (2x – 3)(x – 1)

  • Step #2: Find the critical points.
    • The critical points are

x = 3/2 and x = 1,

which are the roots of the factored quadratic expression.

  • Step #3: Plot the critical points on a number line. Mark x = 3/2 and x = 1 on the number line.

Quadratic Inequalities example

  • Step #4: Test the intervals.

Test the interval x<3/2 by picking x=1 and substituting it into the original inequality:

(2(1)2-5(1)+3)<0,

which is false.

Therefore, the interval x<3/2 does not satisfy the inequality.

Test the interval 3/2<x<1 by picking x=5/4 and substituting it into the original inequality:

(2(5/4)2 – 5(5/4) + 3) < 0,

which is true.

Therefore, the interval 3/2<x<1

Practice Questions

Question 1: Solving the Quadratic Inequality: x2 - 5x + 6 > 0

Answer :


Question 2: Determining the Solution Set for 2x2 - 3x ≤ 1

Answer :

Exam Tips...

  • It’s important to remember that when multiplying or dividing both sides of an inequality by a negative number, we need to reverse the inequality symbol.

Worksheet on Quadratic Inequalities

Question 1: Finding the Values of x that Satisfy -x2 + 4x - 3 < 0



Question 2: Solve the equation: 2x2 - 5x + 2 < 0.



Question 3: Determining the Solution Set for  x2 + 9x + 20 > 0.



Question 4: Finding the Values of x that Satisfy -2x2 + 5x - 2 ≤ 0.



Question 5: Solve the equation: 4x2 - 16x + 15 < 0.