Nth Term of a Linear Sequence | How to Find It with Examples
In this article, we will discuss how to find the nth term of a linear sequence, also known as an arithmetic sequence.
Nth Term of a Linear Sequence
This fundamental concept in mathematics allows you to determine any term in a sequence without listing all the preceding terms.
We will discuss are:
- How to find the nth term of a linear sequence
- Solving various problems related to sequences and series
They are very important in practicing questions for coordinate geometry as well.
Here is one more link to practice a few extra questions: Maths Genie Nth Term of a Linear Sequence Questions
What Is a Linear Sequence?
- A linear sequence is a list of numbers where the difference between any two consecutive terms is always the same.
- This constant difference is known as the common difference.
Example of a Linear Sequence:

Consider the sequence: 2, 5, 8, 11, 14
From 2 to 5: Add 3
From 5 to 8: Add 3
From 8 to 11: Add 3
From 11 to 14: Add 3
Common Difference (A): 3
General Formula for the Nth Term
The general formula to find the nth term of a linear sequence is:
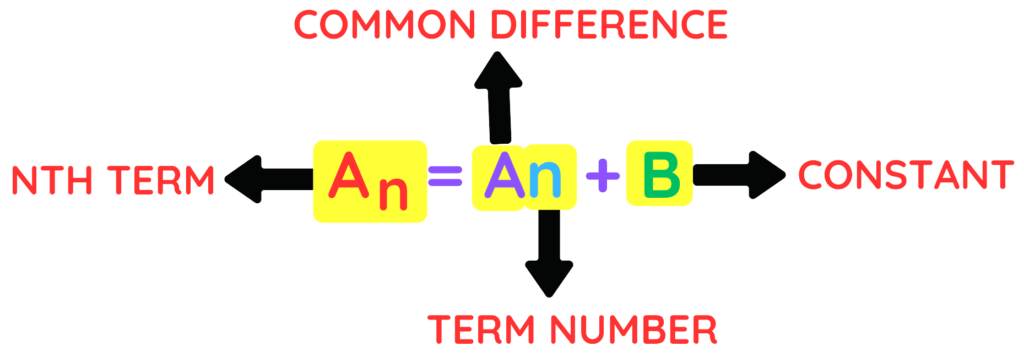
nth term = A × n + B
Where:
- A is the common difference
- B is a constant (the adjustment needed to match the first term)
- n is the term number
Our goal is to find the values of A and B to create a formula that can calculate any term in the sequence.
Steps to Find the Nth Term
To find the general term of a sequence, follow these two main steps:
Step 1: Find the Common Difference (A)
- Subtract any term from the term that follows it.
- Ensure the difference is consistent throughout the sequence.
Step 2: Find the Constant Term (B)
- Use the first term of the sequence.
- Subtract the common difference from the first term: B = First term − A
Solved Example 1
Question: Here are the first 5 terms of an arithmetic sequence.
2, 5, 8, 11, 14
Find an expression, in term of n, for the nth term of sequence and using that find 10th term of the sequence.
Solution:
Given Sequence: 2, 5, 8, 11, 14
Step 1: Find the Common Difference (A)
5 – 2 = 3
8 – 5 = 3
• Common difference A = 3
Step 2: Find the Constant Term (B)
• First term is 2
B = 2 – 3
= −1
Step 3: Nth Term Formula:
nth term = A × n + B
nth term = 3n − 1
Finding a Specific Term
To find the 10th term:
nth term = 3 × 10 − 1
nth term = 29
Solved Example 2
Question: Here are the first 5 terms of an arithmetic sequence.
6, 11, 16, 21, 26
Find an expression, in term of n, for the nth term of sequence and using that find 52nd term of the sequence.
Solution:
Given Sequence: 6, 11, 16, 21, 26
Step 1: Find the Common Difference (A)
11 – 6 = 5
16 – 11 = 5
• Common difference A = 5
Step 2: Find the Constant Term (B)
• First term is 6
B = 6 – 5
= 1
Step 3: Nth Term Formula:
nth term = A × n + B
nth term = 5n + 1
Finding a Specific Term
To find the 52nd term:
nth term = 5 × 52 + 1
nth term = 261
Solved Example 3
Question: Here are the first 5 terms of a number sequence.
3, 7, 11, 15, 19
Write down an expression, in terms of n, for the nth term of this sequence and verify whether 319 is a term in the sequence. You must justify your answer.
Solution:
Given Sequence: 3, 7, 11, 15, 19
Step 1: Find the Common Difference (A)
7 – 3 = 4
11 – 7 = 4
• Common difference A = 4
Step 2: Find the Constant Term (B)
• First term is 3
B = 3 – 4
= -1
Step 3: Nth Term Formula:
nth term = A × n + B
nth term = 4n – 1
To check if 319 is a term in this sequence:
Set Up the Equation
4n − 1 = 319
Solve for n
- Add 1 to both sides:
4n = 320
- Divide both sides by 4:
n = 80
Conclusion
- Since n = 80 is a whole number, 319 is the 80th term of the sequence.
- Therefore, 319 is a term in the sequence.
Note: If n had not been a whole number (e.g., n = 80.5), then 319 would not be a term in the sequence.
Summary of Steps
1. Find the Common Difference (A):
- Subtract consecutive terms to find A.
2. Find the Constant Term (B):
- Subtract the common difference from the first term:
B = First term − A
3. Write the Nth Term Formula:
- Combine A and B into the formula:
nth term = A × n + B
4. Find Any Term in the Sequence:
- Substitute the desired term number (n) into the formula.
Conclusion
Finding the nth term of a linear sequence is a straightforward process once you understand the steps involved:
1. Determine the common difference between the terms.
2. Calculate the constant term by adjusting the first term.
3. Formulate the nth term using the general formula.
4. Apply the formula to find any term in the sequence or verify if a number is part of the sequence.
Practice Questions and Answers on Nth Term of a Linear Sequence
Question 1: Sequence: 4, 9, 14, 19, 24
- Tasks:
- Find the nth term formula.
- Calculate the 30th term.
Question 2: Sequence: 15, 12, 9, 6, 3
- Tasks:
- Find the nth term formula.
- Determine if −12 is a term in the sequence.
Question 3: Sequence: −5, 0, 5, 10, 15
- Tasks:
- Find the nth term formula.
- Find the 25th term.
Question 4: Sequence: 7, 10, 13, 16, 19
- Tasks:
- Find the nth term formula.
- Calculate the 15th term.
Question 5: Sequence: 20, 17, 14, 11, 8
- Tasks:
- Find the nth term formula.
- Determine if −22 is a term in the sequence.
Question 6: Sequence: 1, 4, 7, 10, 13
- Tasks:
- Find the nth term formula.
- Find the 100th term.
Question 7: Sequence: −2, 0, 2, 4, 6
- Tasks:
- Find the nth term formula.
- Calculate the 50th term.
Question 8: Sequence: 100, 95, 90, 85, 80
- Tasks:
- Find the nth term formula.
- Determine if 50 is a term in the sequence.
Question 9: Sequence: 5, 9, 13, 17, 21
- Tasks:
- Find the nth term formula.
- Find the 40th term.
Question 10: Sequence: 50, 47, 44, 41, 38
- Tasks:
- Find the nth term formula.
- Determine if 2 is a term in the sequence.
Solutions
Question 1:
Step 1: Find the Common Difference (A)
9 – 4 = 5
A = 5
Step 2: Find the Constant Term (B)
- First term is 4
B = 4 – 5
= −1
Nth Term Formula
nth term = 5n − 1
Calculate the 30th Term
nth term = 5 × 30 − 1
= 149
Question 2:
Step 1: Find the Common Difference (A)
12 – 15 = −3
A = −3
Step 2: Find the Constant Term (B)
- First term is 15
B = 15 – (−3)
= 18
Nth Term Formula
nth term = −3n + 18
Determine if −12 Is in the Sequence
Set up the equation:
−3n + 18 = −12
Solve for n:
1. Subtract 18 from both sides:
−3n = −30
2. Divide both sides by −3:
n = 10
Conclusion
- Since n = 10 is a whole number, −12 is the 10th term.
- Therefore, −12 is a term in the sequence.
Question 3:
Step 1: Find the Common Difference (A)
0 – (−5) = 5
A = 5
Step 2: Find the Constant Term (B)
- First term is −5
B = (−5) – 5 = −10
Nth Term Formula
nth term = 5n − 10
Find the 25th Term
nth term = 5 × 25 − 10
= 115
Question 4:
Step 1: Find the Common Difference (A)
10 – 7 = 3
A = 3
Step 2: Find the Constant Term (B)
- First term is 7
B = 7 – 3 = 4
Nth Term Formula
nth term = 3n + 4
Calculate the 15th Term
nth term = 3 × 15 + 4
= 49
Question 5:
Step 1: Find the Common Difference (A)
17 – 20 = −3
A = −3
Step 2: Find the Constant Term (B)
- First term is 20
B = 20 – (−3) = 23
Nth Term Formula
nth term = −3n + 23
Determine if −22 Is in the Sequence
Set up the equation:
−3n + 23 = −22
Solve for n:
1. Subtract 23 from both sides:
−3n = −45
2. Divide both sides by −3:
n = 15
Conclusion
- Since n = 15 is a whole number, −22 is the 15th term.
- Therefore, −22 is a term in the sequence.
Question 6:
Step 1: Find the Common Difference (A)
4 – 1 = 3
A = 3
Step 2: Find the Constant Term (B)
- First term is 1
B = 1 – 3 = −2
Nth Term Formula
nth term = 3n − 2
Find the 100th Term
nth term = 3 × 100 − 2
= 298
Question 7:
Step 1: Find the Common Difference (A)
• 0 minus (−2) equals 2
• A = 2
Step 2: Find the Constant Term (B)
- First term is −2
B = (−2) – 2 = −4
Nth Term Formula
nth term = 2n − 4
Calculate the 50th Term
nth term = 2 × 50 − 4
= 96
Question 8:
Step 1: Find the Common Difference (A)
95 – 100 = −5
A = −5
Step 2: Find the Constant Term (B)
- First term is 100
B = 100 – (−5) = 105
Nth Term Formula
nth term = −5n + 105
Determine if 50 Is in the Sequence
Set up the equation:
−5n + 105 = 50
Solve for n:
1. Subtract 105 from both sides:
−5n = −55
2. Divide both sides by −5:
n = 11
Conclusion
- Since n = 11 is a whole number, 50 is the 11th term.
- Therefore, 50 is a term in the sequence.
Question 9:
Step 1: Find the Common Difference (A)
9 – 5 = 4
A = 4
Step 2: Find the Constant Term (B)
- First term is 5
B = 5 – 4 = 1
Nth Term Formula
nth term = 4n + 1
Find the 40th Term
nth term = 4 × 40 + 1
= 161
Question 10:
Step 1: Find the Common Difference (A)
47 – 50 = −3
A = −3
Step 2: Find the Constant Term (B)
- First term is 50
B = 50 – (−3) = 53
Nth Term Formula
nth term = −3n + 53
Determine if 2 Is in the Sequence
Set up the equation:
−3n + 53 = 2
Solve for n:
1. Subtract 53 from both sides:
−3n = −51
2. Divide both sides by −3:
n = 17
Conclusion
- Since n = 17 is a whole number, 2 is the 17th term.
- Therefore, 2 is a term in the sequence.

